In photography we don't know what to expect exactly, so don't we measure the outcome during exposure, which comes with a mean and a standard deviation?
-
-
@JACS has written:@JohnVickers has written:@JACS has written:@JohnVickers has written:
Bernoulli is maybe a bit more specific then "Binomial". But that's maybe getting a bit pedantic.
The way I think about this special case of a compound Poisson process is:
In the base Poisson process, the probability of an arrival in an infinitesimal interval of length dt is 𝝺dt.
In the compound process, each arrival in the base process has a probability p of being accepted.
So in the compound process, the probability of an arrival in an infinitesimal interval of length dt is (𝝺p)dt.
And we have a new Poisson process, with rate parameter 𝝺p.
@JACS has written:This does not explain why it would be a Poisson process.
"the probability of an arrival in an infinitesimal interval of length dt is 𝝺dt" - where 𝝺 is a constant - is the defining characteristic of a (homogeneous) Poisson Process.
"The" is wrong. It is one of them for a continuous Poisson process but I (and we) are not talking about it. There are few more defining properties, one of them is the infinitesimal probability of 0,1, and more arrivals, which is its distribution in that sense. Another one is independent increments. In any case, involving continuous Poisson processes in this case is mudding the waters. Eventually, we will integrate in t anyway, why not assume it done since the beginning.
Quoted message:You could dress that up in measure theory, but that would not change the basic meaning, and would be an obfuscation in this context.
It follows directly from "the probability of an arrival in an infinitesimal interval of length dt is 𝝺dt" that the number of arrivals in any given finite interval has a Poisson distribution.
And from the few more properties needed to be Poisson but this is irrelevant because we already have a Poisson (sometimes called -point-) process. No need to go back in time.
Quoted message:@JACS has written:It just says that its expectation would be 𝝺p.
That's dimensionally incorrect.
The distribution of the number of arrivals in the compound process in a time interval of (finite) duration t is Poisson(𝝺pt).
The time is fixed, of course, and our starting point is a Poisson point process with an expectation 𝝺. We do not care at this point where it comes from, we forget about time. Next, it is randomized additionally by the QE of the pixels.
Quoted message:@JACS has written:One has to start with the probability distribution of a Poisson process and take it from there, if you want to follow this path.
No.
A Poisson process does not, of itself, have any distribution.
See above.
Quoted message:A Poisson distribution can be derived from the (Poisson) arrival process, when considering the arrivals over some (finite) interval.
Maybe there's some misunderstanding about the word "process".
More like which one - the time dependent, roughly speaking, or the discrete one at a fixed time.
Here is an elementary way to derive the Posson characteristics following what I said. At each pixel, we have the probability of n arrivals given by the well known Poisson characteristic P(n,𝝺) with expectation 𝝺. Out of it, we detect k<=n electrons with a binomial probability B(k,n,QE), see the formula for f here. Those are all ways to get k electrons, independent, so we just have to sum up P(n,𝝺)B(k,n,QE) over n>=k. This is a product of discrete measures, BTW. A simple calculation shows this to be P(n,𝝺QE).
Good discussion JACS and John, I learned more about shot noise and its workings in this thread than at any other time in my photographic explorations. I might try to write this up for the less well versed.
-
@JACS has written:
[...]
Here is an elementary way to derive the Posson characteristics following what I said.
At each pixel, we have the probability of n arrivals given by the well known Poisson characteristic P(n,𝝺) with expectation 𝝺.I've explained why there is no need to invoke "well known". The Poisson distribution is a consequence.
Here's a very nice series of short lectures that might help some folk: MIT RES.6-012 Introduction to Probability, Spring 2018
Particularly L22.1..10. Or start earlier if more background is needed. L21.n covers (discrete time) Bernoulli processes.
Fun Fact: The idea of a Poisson process evolved about 100 years after Poisson's death. Poisson himself never studied them.
I think we've done this to death.
-
-
@JohnVickers has written:
I've explained why there is no need to invoke "well known". The Poisson distribution is a consequence.
Everything is a consequence of something else in a way. The (continuous) Poisson process is a consequence of the way light is created in the first place, depending on the source; then you may want to go down to QM to understand why, etc.
-
@JackHogan has written:
I learned more about shot noise and its workings in this thread than at any other time in my photographic explorations.
Ditto.
-
@JACS has written:
We will be arguing next what the meaning of the word "is" is.
Only if someone starts making dogmatic statements about what 'is' means.
-
@JackHogan has written:
In photography we don't know what to expect exactly, so don't we measure the outcome during exposure, which comes with a mean and a standard deviation?
It's always the case in communication that the receiver does not know what is the message, yet the message that the sender sent is the expected value. In the case of a photograph the expected value is a range of brightnesses in the original scene. Shot noise is 'noise' with respect to that because it results in deviation from those expected values. In a scene with a range of brightnesses you can't find the 'expected value' simply by taking the mean.
-
the red queen said in a commanding tone something like "a word means what your make it mean"; I agree that this thread has beeen enlightening.
p.
-
@bobn2 has written:
In a scene with a range of brightnesses you can't find the 'expected value' simply by taking the mean.
You are grossly distorting the meaning of expected value in statistics with this and the other comments you made. If you want to account for variable brightness across the scene (just so that you can keep arguing), you need to introduce two new dimensions but the expected value still exists and is a function of the position as well.
-
@JimKasson has written:
It has always seemed to me strange that the distribution of counted photons is Poisson regardless of the QE of the detector. That goes to Eric's "both" comment.
Yes, Eric Fossum has quite a few pertinent things to say on the DPReview thread: www.dpreview.com/forums/thread/4710831
-
@sybersitizen has written:
Is photon noise inherent in light itself, or is it 'created' by something with which light interacts? Both?
Brief and concise are desirable. Here is a concise answer: Photon noise is "a spatially and temporally random phenomenon described by Bose-Einstein statistics". [1]
But that doesn't answer your questions unless you happen to be familiar with bosons.
The following quote from E. M. Purcell [2] is more informative. I hope you find it brief and concise.
“*Think then, of a stream of wave packets each about c/Δν long, in a random sequence [c = speed of light, v = frequency]. There is a certain probability that two such trains accidentally overlap. When this occurs they interfere and one may find four photons, or none, or something in between as a result. It is proper to speak of interference in this situation because the conditions of the experiment are just such as will ensure that these photons are in the same quantum state. To such interference one may ascribe the ‘abnormal’ density fluctuations in any assemblage of bosons.”
E. M. Purcell 1956, Nature, 178, 1449.
Purcell referred to these density fluctuations as wave noise.
So, according to Purcell.
@sybersitizen has written:"Is photon noise inherent in light itself.
Yes, it is.
@sybersitizen has written:...or is it 'created' by something with which light interacts? Both.
The answer is both. Light waves can interact with each other. When groups of light waves are in superposition (the definition of a wave packet) they interact with each other, constructive and, or destructive wave interference occurs. The Schrödinger equation applies [3] and the result is an amplitude (i.e. non-classical) probability distribution (Purcell's "abnormal density fluctuations") in photoelectron production.
This means the distribution of photoelectron production we observe in digital photographs as noise is linked to the same mathematics that describes electron density in atoms. Of course, photoelectron production and electron probability density in atoms are completely dissimilar.
I have now strayed from brief and concise.
But Purcell's answer to your questions means speculation about fluctuations in photon creation are unnecessary. Wave packet interference occurs after photon creation. Fluctuations in photon arrival are unnecessary as well. Arrival is irrelevant since the fluctuations are amplitude density fluctuations.
Purcell's answer certainly means invoking Newtonian physics analogies such as raindrops or particles falling into buckets do not apply to photon noise.
1. James Janesick "Photon Noise", SPIE Press, 2007, p21.
2. According to Wikopedia: "Edward Mills Purcell (August 30, 1912 – March 7, 1997) was an American physicist who shared the 1952 Nobel Prize for Physics for his independent discovery (published 1946) of nuclear magnetic resonance in liquids and in solids.")
3. The Schrödinger Equation, Terence Tao, Department of Mathematics, UCLA, Los Angeles CA (see footnote 2)
4. A. A. Clerk, M. H. Devoret, S. M. Girvin, Florian Marquardt, and R. J. Schoelkopf Introduction to Quantum Noise, Measurement and Amplification Rev. Mod. Phys. 82, 1155 – Published 15 April 2010. Eqn 2.1 -
@AShortUserName has written:
Think then, of a stream of wave packets each about c/Δν long, in a random sequence [c = speed of light, v = frequency]
This is a common description, but I have a problem with it since watching this video called "How big is a photon?":
www.youtube.com/watch?v=SDtAh9IwG-I&tHe sets up an experiment where he splits the light from a HeNe laser and then combines the beams to get interference. The light intensity is sow low that statistically there is only one photon in the beam paths at at any given time, and the lengths of the two paths are also different. If photons are wave packets localized in space, and if we increase the path length difference enough, we should see a reduction in the interference pattern, but this doesn't seem to be the case. The length difference doesn't matter at all. If the size of these packets are infinite, are they really "packets"?
Instead he argues that light is an electromagnetic field which is not quantized. Quantization happens when we interact with the field, and the probability of detection of a photon is proportional to the intensity of the field.
So there are no photons. Only localized field interactions which we call photons. I'm not a phycisist so I don't know if this is correct, but to me it is at least intuitive. It makes it very easy to explain the experiment in the video. Does this also apply to other particles? I have no clue, but it sounds similar to the question in quantum mechanics "does the world exist when we're not looking?".
-
@AShortUserName has written:
“*Think then, of a stream of wave packets each about c/Δν long, in a random sequence [c = speed of light, v = frequency]. There is a certain probability that two such trains accidentally overlap. When this occurs they interfere and one may find four photons, or none, or something in between as a result. It is proper to speak of interference in this situation because the conditions of the experiment are just such as will ensure that these photons are in the same quantum state. To such interference one may ascribe the ‘abnormal’ density fluctuations in any assemblage of bosons.”
Oh, no. This type of "wave noise" is largely irrelevant for photography.
-
@JohnA has written:@AShortUserName has written:
Think then, of a stream of wave packets each about c/Δν long, in a random sequence [c = speed of light, v = frequency]
This is a common description, but I have a problem with it since watching this video called "How big is a photon?":
www.youtube.com/watch?v=SDtAh9IwG-I&tSee the link posted here: www.dpreview.com/forums/thread/4710831?page=3#forum-post-67026232. The wave packet theory is in the past, it seems.
-
@AShortUserName has written:
Purcell's answer certainly means invoking Newtonian physics analogies such as raindrops or particles falling into buckets do not apply to photon noise.
Interesting post, I can't comment on wave/particle duality because I understand the issue has not been satisfactorily resolved yet. But with regards to Purcell's comment above, perhaps they are not Newtonian physics analogies, rather observations that can be explained statistically at pixel scale thanks to Planck/Einstein and others.
For instance if one were to assume that the raindrops falling in one bucket arrived at a certain rate but with random timing without collisions, they would arrive with the observed Poisson distribution. Just like photons from a source landing on a pixel's photodiode. And we can actually measure the relative counts.
Jack
-
@AShortUserName has written:
The answer is both. Light waves can interact with each other. When groups of light waves are in superposition (the definition of a wave packet) they interact with each other, constructive and, or destructive wave interference occurs. The Schrödinger equation applies [3] and the result is an amplitude (i.e. non-classical) probability distribution (Purcell's "abnormal density fluctuations") in photoelectron production.
Jack's post above made me take another look at this post of AShortUserName. There is so much misinformation even in that paragraph, I do not even know where to start. "Light waves can interact with each other" - not in the linear model mentioned later. "When groups of light waves are in superposition (the definition of a wave packet)" : no, this is not a definition of a wave packet, you are thinking of a superposition of wave packets, as Tao says. "They interact with each other": actually, a superposition means linearity (a sum) which is on the opposite side of interaction. "The Schrödinger equation applies [3]": it does not, it is the QED for photons but even if we forget that, the (linear) Schrödinger equation excludes interaction, and we do have a superposition. "The result is an amplitude (i.e. non-classical) probability distribution (Purcell's "abnormal density fluctuations") in photoelectron production": this is not even wrong, it just does not mean anything.
-
@JohnA has written:
This is a common description, but I have a problem with it since watching this video called "How big is a photon?":
www.youtube.com/watch?v=SDtAh9IwG-I&tA stimulating video. However, he loses me when he says
Quoted message:[...] what we do [...] is gathering statistical information about the spatial probability of detecting a photon somewhere. And this probability itself, like the field, is not quantized.
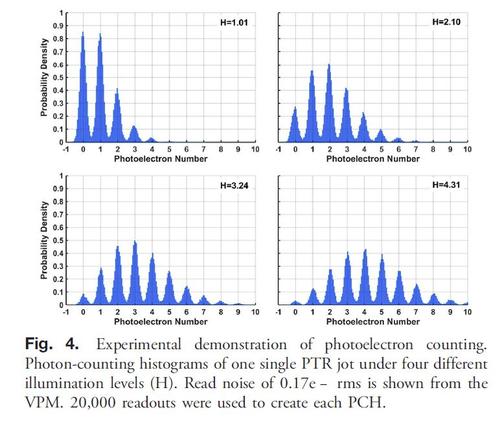
On the contrary, the spatial/time probability of detecting a photon is indeed quantized, as we have been able to observe for quite some time once we got clean detectors, see for instance recently Eric Fossum's QIS work:.
As he explains, the Gaussian looking shapes around each peak are the result of the convolution of the detector's read noise with impulses representing the discrete Poisson distribution of actual detected counts. As detector read noise gets smaller and smaller, the resulting probability distribution tends to the discrete Poisson probability mass function.
So if the resulting probability (light*detection) is quantized with a Poisson distribution, what can we say about the field from the source?
We know from an earlier post that Poisson*Binomial = Poisson, therefore the field being quantized would match observation.
What class of continuous field probability distributions times Binomial detection would result in a Poisson distribution out?
Jack