Here's my question: aside from changing the position or focal point of the camera (and/or movements), how does one change the perspective of the photo for a given lens? That is, is it possible to move the camera and take another photo and get a photo with the same perspective?
-
-
HAND.
-
If you move the camera, then the photo will change. It is not the same photo. I think everyone agrees on that, it is not in dispute. I am not sure where this is going?
-
If I move closer, or further away to the building in the picture below, then the prospective effect on the building ( our subject) will change.
We can deduce this from Brunelleschi's experiment.
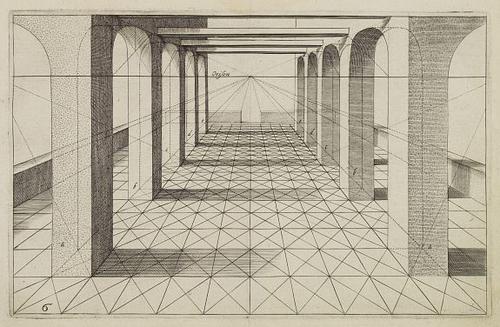
But for the scene as a whole, in the case below, you tell me. The angle of view of the camera lens remains the same and the perspective effect on the tiled floor remains the same as I move forward.
-
@NCV has written:
We can deduce this from Brunelleschi's experiment.
Thanks for your posts, although I confess to not being sure exactly what you are saying.
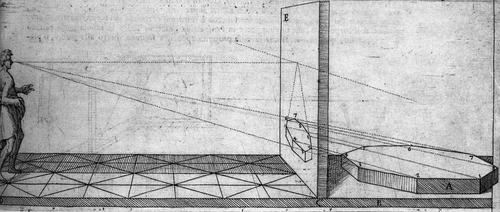
I rather like the following diagram from Jacopo Barozzi da Vignola (1583) in which a drawing or painting is imagined as transparent and the lines of sight are shown in more detail. When viewed from the centre of perspective, every point in the 2D image lines up exactly with the corresponding point on the 3D object. The position of the viewer's eye is critical.
It is obvious that if the viewer moves either closer to the image or further away, then the image will be seen with a different perspective in which points on the image cannot all line up with the corresponding points on the object.
- from WikimediaThis diagram works equally well if we imagine that the image is a photograph on a transparent medium. The viewer needs to be at exactly the position of the camera when the photo was taken. It should then be possible to position the image so that everything in the image lines up exactly with the real scene behind it. The viewer's eye is then at the centre of perspective of the photograph.
-
@TomAxford has written:@JACS has written:
As a scientist myself, I object those science references. There is no fallacy, the online tutorials are mostly right on this for people not speaking in precise terms. One can add a bit to it, put it in a more general perspective (pun intended) but calling it incorrect science is over the top.
It is a fallacy to claim that perspective is determined solely by camera position.
Perspective depends on both the camera position (relative to the scene) and the viewer's position (relative to the image). This is explained clearly in the Manual of Photography by Jacobson et. al. (7th edition, 1978), and other places. It is incorrect science when people claiming to explain perspective distortion fail to mention the significance of the viewer's position relative to the image.
If you claim that perspective depends only on camera position, then the onus is on you to prove it.
The viewers position has NOTHING to do with the perspective of the image they are viewing, it changes the perspective they see, but not the perspective of the image.
You may as well claim an image is underexposed because the viewer has the lights off!
-
@petrochemist has written:
The viewers position has NOTHING to do with the perspective of the image they are viewing, it changes the perspective they see, but not the perspective of the image.
Obviously.
Perspective distortions such as telephoto compression do not exist in the image per se, but they may exist in our view of the image.
-
@TomAxford has written:@petrochemist has written:
The viewers position has NOTHING to do with the perspective of the image they are viewing, it changes the perspective they see, but not the perspective of the image.
Obviously.
Perspective distortions such as telephoto compression do not exist in the image per se, but they may exist in our view of the image.
Actually 'Telephoto compression' is in the image - it is exactly the distance related perspective discussed above. It's not something magical to the lens cropping or enlarging a normal image from the same position will show just the same perspective.
-
@petrochemist has written:
Actually 'Telephoto compression' is in the image - it is exactly the distance related perspective discussed above. It's not something magical to the lens cropping or enlarging a normal image from the same position will show just the same perspective.
So, you are saying that telephoto compression is in the image itself and does not depend in any way on how that image is viewed, is that correct?
If that is so, please describe precisely how you would determine that one image has telephoto compression while another does not. And how much telephoto compression does it contain, can you quantify it?
I don't think your theory will survive any close scrutiny, but let's see what you can come up with.
-
For me as a photographer, the only thing that matters is the "feel" of the used 'perspective distortion'. When I want a flat, correct 'look', I will take a long lens; when I want a 'close, spectacular' look, I will use a short lens.
-
@Diederik has written:
For me as a photographer, the only thing that matters is the "feel" of the used 'perspective distortion'. When I want a flat, correct 'look', I will take a long lens; when I want a 'close, spectacular' look, I will use a short lens.
For me it's often decided by how close I can get to the subject. For air shows & motorsports close is rarely an option, and for real estate a wide angle can be need to get enough in - making the rooms look big is a side advantage 😁
-
@TomAxford has written:@petrochemist has written:
Actually 'Telephoto compression' is in the image - it is exactly the distance related perspective discussed above. It's not something magical to the lens cropping or enlarging a normal image from the same position will show just the same perspective.
So, you are saying that telephoto compression is in the image itself and does not depend in any way on how that image is viewed, is that correct?
If that is so, please describe precisely how you would determine that one image has telephoto compression while another does not. And how much telephoto compression does it contain, can you quantify it?
I don't think your theory will survive any close scrutiny, but let's see what you can come up with.
The 'compression' - the perspective - is defined by relative sizes of objects in the frame and their relative positions. That's solely defined by the camera position and distance to the objects. It doesn't depend on the distance between viewer's eyes and a printed photo.
I know this argument has been going around in circles for many months now - but nothing can change the fact stated above - maybe a different universe with different physics and optics?..
-
@Quarkcharmed has written:@TomAxford has written:@petrochemist has written:
Actually 'Telephoto compression' is in the image - it is exactly the distance related perspective discussed above. It's not something magical to the lens cropping or enlarging a normal image from the same position will show just the same perspective.
So, you are saying that telephoto compression is in the image itself and does not depend in any way on how that image is viewed, is that correct?
If that is so, please describe precisely how you would determine that one image has telephoto compression while another does not. And how much telephoto compression does it contain, can you quantify it?
I don't think your theory will survive any close scrutiny, but let's see what you can come up with.
The 'compression' - the perspective - is defined by relative sizes of objects in the frame and their relative positions. That's solely defined by the camera position and distance to the objects. It doesn't depend on the distance between viewer's eyes and a printed photo.
I know this argument has been going around in circles for many months now - but nothing can change the fact stated above - maybe a different universe with different physics and optics?..
I think it's helpful to refer (again) to what Adams actually wrote about what happens with the image recorded in the camera vs. what happens when the resulting image is viewed:
He calls the first thing the 'true perspective' and calls the second thing the 'impression of the perspective' or the 'perspective effect'. That works for me.
-
@Quarkcharmed has written:@TomAxford has written:@petrochemist has written:
Actually 'Telephoto compression' is in the image - it is exactly the distance related perspective discussed above. It's not something magical to the lens cropping or enlarging a normal image from the same position will show just the same perspective.
So, you are saying that telephoto compression is in the image itself and does not depend in any way on how that image is viewed, is that correct?
If that is so, please describe precisely how you would determine that one image has telephoto compression while another does not. And how much telephoto compression does it contain, can you quantify it?
I don't think your theory will survive any close scrutiny, but let's see what you can come up with.
The 'compression' - the perspective - is defined by relative sizes of objects in the frame and their relative positions. That's solely defined by the camera position and distance to the objects. It doesn't depend on the distance between viewer's eyes and a printed photo.
I know this argument has been going around in circles for many months now - but nothing can change the fact stated above - maybe a different universe with different physics and optics?..
Well, maybe that universe with different physics and optics exists solely in my, and a few others', heads, as it is clear enough and observable enough to me.
-
@TomAxford has written:@petrochemist has written:
Actually 'Telephoto compression' is in the image - it is exactly the distance related perspective discussed above. It's not something magical to the lens cropping or enlarging a normal image from the same position will show just the same perspective.
So, you are saying that telephoto compression is in the image itself and does not depend in any way on how that image is viewed, is that correct?
If that is so, please describe precisely how you would determine that one image has telephoto compression while another does not. And how much telephoto compression does it contain, can you quantify it?
I don't think your theory will survive any close scrutiny, but let's see what you can come up with.
I wouldn't have any desire to determine it let alone do it precisely.
If I needed to do it as an exercise I would shoot a series of items of similar height, in a reasonably straight line & separated by regular distances (Telegraph poles or fence posts are good options) comparing the relative heights of these on the the image would show the perspective.If you are standing right by one telegraph pole, the next will be x feet away, then 2x, 3x, 4x etc.
This makes the second in view twice as far as the first (and so the second has half the apparent height). A wide angle may be needed to get all of the nearest pole in .
The third is 1.5 times as far as the second (3x/2x) so has 66% of the apparent height of the second. This could be the typical view of a normal lens
By the time you've got to the tenth pole with distances of 9x & 10x the apparent height of adjacent poles only differs by about 10%. This might be the sort of distance typically seen with a telephoto lens, (it depends on the focal length & sensor size or how telephoto the lens is).
(Technically a telephoto is actually one that is physically shorter than it's focal length, but here I'm working with the more common understanding of telephoto as simply a long focal length lens).Accurately measuring the height of the tenth pole in the series might be a little challenging, but there are ways to do it reasonably well. You will see the same relationship between poles 9 & 10 on a wide angle lens as with the telephoto, its just the telephoto doesn't give you the height of the nearer poles.
-
@Bryan has written:@Quarkcharmed has written:@TomAxford has written:@petrochemist has written:
Actually 'Telephoto compression' is in the image - it is exactly the distance related perspective discussed above. It's not something magical to the lens cropping or enlarging a normal image from the same position will show just the same perspective.
So, you are saying that telephoto compression is in the image itself and does not depend in any way on how that image is viewed, is that correct?
If that is so, please describe precisely how you would determine that one image has telephoto compression while another does not. And how much telephoto compression does it contain, can you quantify it?
I don't think your theory will survive any close scrutiny, but let's see what you can come up with.
The 'compression' - the perspective - is defined by relative sizes of objects in the frame and their relative positions. That's solely defined by the camera position and distance to the objects. It doesn't depend on the distance between viewer's eyes and a printed photo.
I know this argument has been going around in circles for many months now - but nothing can change the fact stated above - maybe a different universe with different physics and optics?..
Well, maybe that universe with different physics and optics exists solely in my, and a few others', heads, as it is clear enough and observable enough to me.
What is observable exactly? You mean relative sizes and positions of objects in the frame depend on, errm, imagination/perception when viewing a photo?
-
@sybersitizen has written:
He calls the first thing the 'true perspective' and calls the second thing the 'impression of the perspective' or the 'perspective effect'. That works for me.
This is a reasonable and helpful division. I can control the "true perspective" of the camera by choosing where to move the camera (and in some cases, by moving elements in the scene) for creative impact, then combine that with the desired focal length to ensure my subjects are the intended size in the frame. By and large, I can't control how people choose to view the output. If someone really wants to get their eyeball an inch away from a print for their viewing pleasure, or walk across the room and squint at the distance, I can't really stop them. In my experience, most people view prints/screens from a relatively narrow range of distances based on what's comfortable for them, and thus will see the effects of my camera/subject placement as per my creative intent.
-
@petrochemist has written:@TomAxford has written:@petrochemist has written:
Actually 'Telephoto compression' is in the image - it is exactly the distance related perspective discussed above. It's not something magical to the lens cropping or enlarging a normal image from the same position will show just the same perspective.
So, you are saying that telephoto compression is in the image itself and does not depend in any way on how that image is viewed, is that correct?
If that is so, please describe precisely how you would determine that one image has telephoto compression while another does not. And how much telephoto compression does it contain, can you quantify it?
I don't think your theory will survive any close scrutiny, but let's see what you can come up with.
I wouldn't have any desire to determine it let alone do it precisely.
If I needed to do it as an exercise I would shoot a series of items of similar height, in a reasonably straight line & separated by regular distances (Telegraph poles or fence posts are good options) comparing the relative heights of these on the the image would show the perspective.If you are standing right by one telegraph pole, the next will be x feet away, then 2x, 3x, 4x etc.
This makes the second in view twice as far as the first (and so the second has half the apparent height). A wide angle may be needed to get all of the nearest pole in .
The third is 1.5 times as far as the second (3x/2x) so has 66% of the apparent height of the second. This could be the typical view of a normal lens
By the time you've got to the tenth pole with distances of 9x & 10x the apparent height of adjacent poles only differs by about 10%. This might be the sort of distance typically seen with a telephoto lens, (it depends on the focal length & sensor size or how telephoto the lens is).
(Technically a telephoto is actually one that is physically shorter than it's focal length, but here I'm working with the more common understanding of telephoto as simply a long focal length lens).Accurately measuring the height of the tenth pole in the series might be a little challenging, but there are ways to do it reasonably well. You will see the same relationship between poles 9 & 10 on a wide angle lens as with the telephoto, its just the telephoto doesn't give you the height of the nearer poles.
The question the OP is asking here here can be simplified with an example. He is interested in the perspective effect of a print due to viewing distance.
So for example, I view a fisheye shot from a very close distance, the perspective will appear normal. I think this is what the Op is getting at.
Of course we all tend to view prints and screens in certain range of distances between 1m an 30cm, in most cases.